Magnets and Induction

Ferromagnetism

Ferromagnetism is a property of some materials which allows it to be magnetised and to create its own magnetic field.

Each particle in a ferromagnetic material has a magnetic dipole - meaning it has a north and south pole. When the material is unmagnetised, these dipoles are scattered in random orientations, causing their individually weak magnetic fields to cancel each other out in a sea of noise. Magnetisation is the process of orienting these dipoles to point in the same direction, creating a significant overall magnetic field. This can be achieved by exposing the material to a strong magnetic field and letting the atoms move around, for example by heating it up. Similarly, demagnetisation happens when the dipole alignments become scattered again.

Magnetic Induction

Confusing terms!

Magnetic induction and electromagnetic induction, despite the similarity in the terms, are not the same! Make sure you know the difference ~~and blame the SQA for the confusing terminology used~~.

Magnetic induction ( $B$ ) is a vector quantity representing the magnitude and direction of a magnetic field at a point. It is measured in teslas ( $T$ ).

Magnetic induction is the magnetic field equivalent to electric field strength for electric fields and gravitational field strength for gravitational fields. However, it cannot be expressed as elegantly as "force per unit charge" or "force per unit mass" respectively, as there is no such thing as a "unit magnetic pole".

Magnetic field around a wire

Formula

$B = \frac{μ_{0} I}{2 π r}$

Variable Key

$B$ is the magnetic induction, in teslas.
$μ_{0}$ is the permeability of free space constant.
$I$ is the current through the wire, in amperes.
$r$ is the distance from the wire, in metres.

Force on a wire

Formula

$F = I l B \sin θ$

Variable Key

$F$ is the force acting on the wire, in newtons.
$I$ is the current through the wire, in amperes.
$l$ is the length of wire in the field, in metres.
$B$ is the magnetic induction, in teslas.
$θ$ is the angle between the current and magnetic field directions.

Force on a charged particle

Formula

$F = q v B$

Variable Key

$F$ is the force acting on the particle, in newtons.
$q$ is the charge of the particle, in coulombs.
$v$ is the velocity of the particle, in metres per second.
$B$ is the magnetic induction, in teslas.

Solenoids

A solenoid is simply a coil of wire. When a direct current passes through the coil, the magnetic induction around each coil adds up to produce a resultant magnetic field, letting the solenoid behave similarly to a permanent magnet.

Solenoids are most commonly used in electromagnets, which allows it to attract ferromagnetic materials when a current passes through it.

Electromagnetic Induction

Confusing terms!

From Fleming's Right-Hand Rule, we already know that when a current moves through a magnetic field, it experiences a force. However, the converse is also true - if we apply a force to move charges through a magnetic field, that will produce a current. This phenomenon is known as electromagnetic induction.

Inductors

An inductor, similar to a solenoid, is a coil of wire. Inductors resist changes in current through a process known as self-inductance.

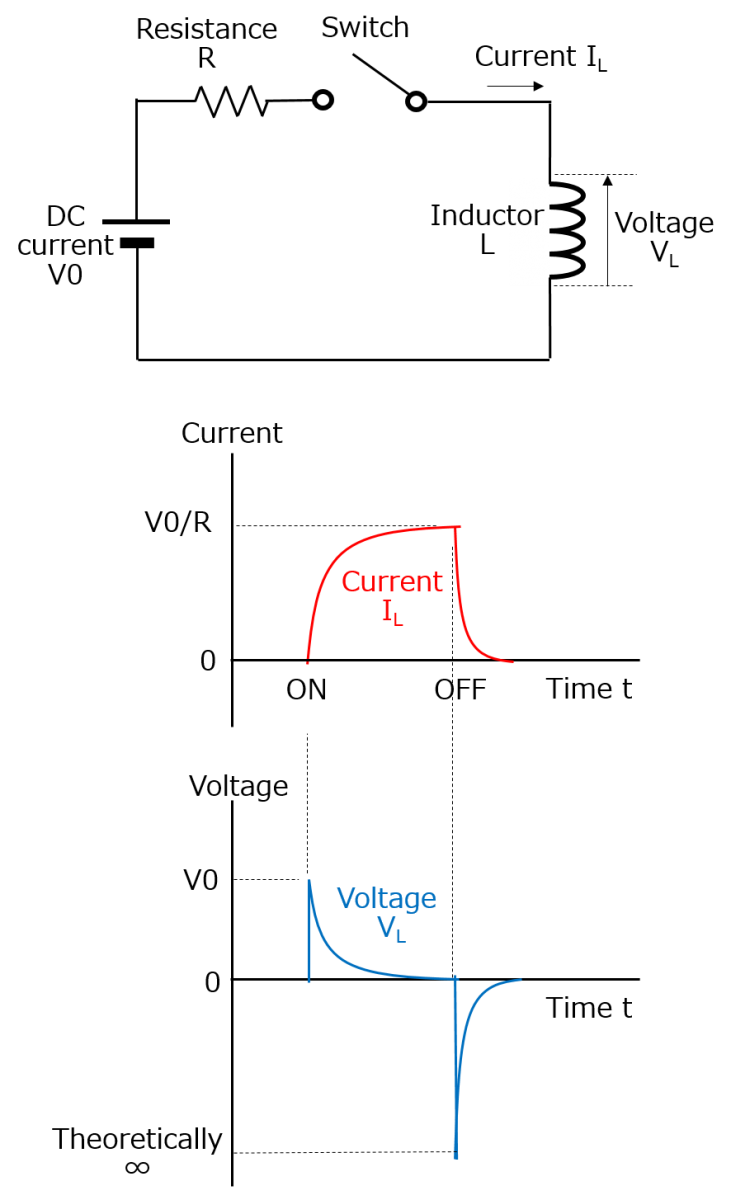
The "strength" of an inductor is known as its inductance ( $L$ ), and is measured in henrys or henries ( $H$ ).

Self-inductance

An inductor works as follows:

When a current starts flowing through the inductor, it induces a magnetic field.
When the magnetic field changes, it induces an electromotive force (EMF).
This back EMF opposes the initial current, which is called self-inductance.

Back EMF

Back EMF, also known as counter-electromotive force, is the electromotive force which opposes the current as a result of self-inductance, as shown above.

Lenz's Law

The relationship between the inductance of an inductor and its induced back EMF is defined by Lenz's Law.

Formula

$ε = - L \frac{d I}{d t}$

Variable Key

$ε$ is the back EMF, in amperes.
$L$ is the inductance of the inductor, in henrys.
$\frac{d I}{d t}$ is the change in current of the EMF, in amperes.

Energy in Inductors

We already know that an inductor induces a back EMF to oppose a change in current. This means that work is done in producing this back EMF current. This energy is stored in the magnetic field itself produced by the inductor - and when the circuit is broken, this energy can be released in a short burst.

The formula for energy stored in an inductor is similar to the equation for kinetic energy:

Formula

$E = \frac{1}{2} L I^{2}$

Variable Key

$E$ is the energy stored in the magnetic field of an inductor, in joules.
$L$ is the inductance of the inductor, in henrys.
$I$ is the current through the inductor, in amperes.